Exactly How Much Wind Correction Should be used on the Outbound Leg of a Hold?

Common rules of thumb when trying to determine how much crosswind correction should be used on the outbound leg of a hold include tripling the wind correction needed on the inbound leg and doubling the wind correction needed on the inbound leg.

Both these rules are useful, but neither is precise enough to be used in all situations.

In order to determine exactly what multiple of inbound wind correction to use on the outbound leg, the dynamical equations for an aircraft in a hold were determined and solved (the math is shown at the end of this post).

The results are displayed in several graphical formats below.

The first graphical representation of required wind correction is in the below graph. In the below graph, when the term headwind or tailwind is used it always means that the headwind/tailwind is on the inbound leg.

Click to Enlarge

It's interesting to note that as wind velocity approaches zero the required multiple approaches exactly 3, so the 3x inbound correction rule of thumb works well in light winds. Also of note, a headwind has a surprisingly large effect on the multiple required when combined with a crosswind.

The second graphical format is the below video which gives exact answers to to the question of required crosswind correction on the outbound leg for all possible integer valued wind directions and a wide range of wind velocities.

The final format is the below .gif which shows some of the same data as the videos, but in a format that is easier to share.

For the numerically inclined, a complete description of the problem and the equations used is given below.

FULL EXPLANATION OF THE PROBLEM AND EQUATIONS USED

Introduction

When operating an aircraft in instrument meteorological conditions (IMC), air traffic control will often require pilots to perform a maneuver called a “hold.” This maneuver requires the pilot to fly in a race-track shaped pattern in the sky as shown below:

There are a number of FAA specified goals when flying a hold, for this paper the relevant goals are:

1. Making the inbound leg exactly 1 minute in length or 1.5 minutes if above 14,000 feet

2. After completing the turn from outbound leg to inbound leg, the aircraft should be tracking exactly towards the station (the station is represented by a greenish triangle above).

3. Constant indicated airspeed

4. When turning, the angular rate of turn should be exactly 3 degrees per second

5. Constant Altitude

This maneuver is very simple in no-wind conditions, but, when there is a crosswind or headwind/tailwind or some combination thereof, achieving goals 1 and 2 can become difficult. When there is a crosswind, complying with goals 1 and 2 distorts the racetrack shape into a vaguely egg-shaped pattern as shown below:

Before continuing, it’s important to understand the difference between heading and course. Heading is the direction that the aircraft is pointed, an aircraft sitting on the ground will have a heading, it will be pointed in some direction. Course is the direction that an aircraft is traveling over the ground, it is the direction that a GPS device would tell you you are moving in if you are flying a GPS-equipped aircraft, a stationary aircraft on the ground does not have a course because it is not moving in any direction. If there is wind, there will usually be a difference between heading and course, the graphic below attempts to further clarify the difference between these two quantities.

The technique that pilots use to achieve goal 2 when holding is to note the difference between course and heading when flying on the *inbound* leg of the hold, this difference is called the “wind correction angle.” Some multiple of this wind correction angle is then added to or subtracted from the aircraft’s no-wind heading when on the *outbound* leg. I’ve never met a pilot that knew exactly what this multiple should be, in undergrad I did some rough calculations and discovered that the multiple increases when the wind correction angle decreases and vice versa, I forget the exact equations I used but the result was that a multiple of 3 should be used when the wind correction angle is 5 degrees, 2.7 should be used when the angle is 10 degrees and 2 should be used when the angle is 20 degrees. If successful in finding the exact formula, I will find out how close these initial calculations were.

The technique that pilots use to achieve goal 1 is to vary the amount of time spent flying on the outbound leg, in no wind conditions the outbound leg is flown for exactly 1:00… But if there is a tailwind when on the inbound leg, more than one minute must be spent on the outbound and if there is a headwind on the inbound leg, less than one minute must be spent on the outbound leg. The rule of thumb used by pilots is to first fly one lap in the hold and add any missing time on the first inbound leg to the outbound leg or subtract any excess time on the inbound leg from the outbound leg. So if the first inbound leg took 1:10 the next outbound leg would be flown for 0:50. This method is obviously inexact as it breaks down in extreme conditions. For example, if on the inbound lag you had a headwind equal to 66.7% of your velocity and you started at a distance that in no wind conditions would result in the time being one minute it would take you 3 minutes to reach the station. In this case the excess time would be 2:00… but if you subtract 2:00 from the normal 1:00 outbound leg you get a negative time value... which is nonsensical.

In this post I will attempt to find an equation that gives the precise multiple that should be used to achieve goal 2 and the precise duration to be flown on the outbound leg to achieve goal 1. I will do this by finding the equation of motion for each of the 4 segments of the hold as functions of wind velocity and heading (with true airspeed held constant). These equations will then be solved for when goals 1 and 2 are met.

Preliminary Calculations

To start we need to find the exact relationship between rate of turn (which must be 3º/second) and bank angle. I will derive this relationship here starting from the equation for normal acceleration in the tangential-normal coordinate system and the equation for radius of turn as a function of bank angle, which will itself be derived from equations for centripetal force and acceleration. Wind is assumed to be constant in these calculations.

1. Finding the Radius of Turn vs. Bank Angle relationship

The formula for centripetal force is: mv^2/r, We are constrained to level flight per goal #5.

Setting the normal forces (the horizontal component of lift) to equal centripetal force we get:

mv^2/r = mgtan(θb)

r = v^2/gtan(θb) (1)

Where r is radius of turn

2. Finding the angle of bank which yields a 3 degrees per second rate of turn.

3 deg/second * pi/180 radians/degree = .05236 radians/second = dθb/dt

In the tangential-normal coordinate system:

F = ma

a = F/m

a = r (dθb/dt)^2 = F/m (2)

Substituting equation 1 into equation 2 and using mgtan(θb)for F:

(v^2/gtan(θb))(.05236)^2 = mgtan(θb)/m

v^2/g2*.00274155=tan^2(θb)

tan(θb) = v/g*.05235983

θb = tan-1(v/g*.05235983)

Using 9.80m/s2 for g and and converting m/s to knots using the following conversion:

[6076ft/nautical mile]/[3600s/hr*3.28ft/m]

θb = tan-1((v/9.80)*[.05235983*6076/(3600*3.28)])

θb = tan-1(.00274925v) (3)

Wind does not affect TAS so in all turns for this problem the angle of bank will be:

tan-1(.00274925*100KTAS)= 15.372 degrees or .26829 radians

Setting up the Problem

There are three parts of the hold which are variable and one which is fixed:

Segment 1 is variable, a constant 15.372 degree bank angle (assuming 100KTAS as in the example) will be held until such a time as the aircraft arrives at the desired heading for segment 2. In this problem we will assume that bank changes occur instantaneously though in reality changing bank from 15 degrees to 0 degrees takes about 2 seconds. Here we will try to determine the equation of motion for this portion of the hold in cartesian coordinates. For this problem we will set the zero degrees line to be parallel to the y axis as shown below, Additionally, because left turns in the hold are used in this example (and also in keeping with mathematical convention) *we will make angular displacement increase when traveling counterclockwise* as shown below, this is non-standard in aviation and could lead to confusion if not noted.

Acceleration here is all in the normal direction so the normal-tangential equation for acceleration:

a = r (dθ/dt)^2 + r(d2θ/dt^2)

becomes

a = r (dθ/dt)^2

The integral of this equation is velocity which is:

v= r(dθ/dt) +c1

In this case c1 is wind velocity, for this project we will use the variable w for wind velocity.

Integrating the above equation gives us position, here t1 represents the elapsed time in the first segment.

(x,y)= rθ +wt1 +c2

Here c2 represents our initial position, we will define the origin to be exactly at the station, therefore c2 is equal to the vector distance from the center of the turn to the station. The center of the turn is located at a distance equal to r in the direction (d) 90° left of heading, where heading is 0° + WCA therefore, d = 90° + WCA and c2 = rsin(WCA)i - rcos(WCA)j and heading at any given time will equal initial wind correction angle (WCA) plus θ degrees where θ = 3t,

Initial wind correction angle is:

WCA = sin-1((wj)/(vaircraft-wi))

Because (dθ/dt) is a constant 3deg/s we can make this equation a cartesian function of time by substituting rθ for rcos(3t1)j - rsin(3t1)i and c2 for rsin(WCA)i - rcos(WCA)j:

(x,y)= rcos(3t1 +WCA)j - rsin(3t1 + WCA)i +wt1 + rsin(WCA)i -rcos(WCA)j (4) 

Segment 2 is variable, desired heading and temporal duration vary with wind conditions.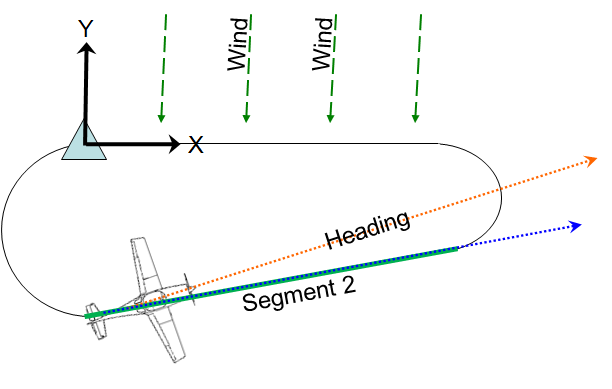 

There is no acceleration during this leg, therefore: a = 0

Taking the integral:

v = c1

Where c1 is equal to aircraft velocity vaircraft plus wind velocity w. The magnitude of the aircraft’s velocity is given as a state variable but the direction is determined by the duration for which segment 1 is flown, we will call this duration variable t1f, therefore:

H2 = 3t1f + sin-1(wj/vaircraft)

H2 = 3t1f + WCA (5)

and velocity can be stated as:

v = -vcos(3t1f + WCA)j + vsin(3t1f  + WCA)i + w

Taking the integral to get position:

(x,y) = -vt2cos(3t1f  +WCA)j - vt2sin(3t1f  + WCA)i + wt2 + c2

Where t2 is the duration for which the second leg has been flown, c2 here represents the starting position which is the final position in segment 1 which is:

(x,y)= rcos(3t1f)j -rsin(3t1f)i +wt1f -rsin(WCA)i -rcos(WCA)j

To simplify equations we will assign this expression the variable p1 therefore the equation for position for segment 2 is:

(x,y) = -vt2fsin(3t1f + WCA)j - vt2fcos(3t1f + WCA)i + wt2 + p1 (6)

Segment 3 has constant bank angle and variable duration, it ends when the desired inbound heading is reached

This segment is identical to segment 1 except for the beginning and end points and the degrees turned through, starting with the equation of motion:

a = r (dθ/dt)^2

The integral of this equation is velocity which is:

v= r(dθ/dt) +c1

In this case c1 is wind velocity, for this project we will use the same variable w for wind.

Integrating the above equation gives us position, here t3 represents the elapsed time in the third segment.

(x,y)= rθ +wt3 +c2

The center of the turn is located at a distance equal to r in the direction (d2) 90° left of heading, where heading is H2 = WCA + t1f3°/s therefore, d2 = 90° + WCA + t1f3°/s and:

(x,y)= rcos(3t3 +H2)j - rsin(3t3 + H2)i +wt3 + c2

and c2 is equal to the position (p2) at the end of segment 2 plus the distance from that position to the center of the circle which is being turned:

c2 = p2 + rd2

To simplify equations we will assign this expression the variable p2 therefore the equation for position for segment 3 is:

(x,y)= rcos(3t3 +H2)j - rsin(3t3 + H2)i +wt3 + p2 + rd2(7)

Segment 4 is fixed, it must be flown for exactly 1 minute, because of this we know exactly where it must start and what direction it must be flown in, the direction is 090° and the distance is (vaircrafti + wi)/60min/hr which is equal to:

(x,y) = 0j + vcos(WCA)i + wi (8)

We will refer to this position as p3 for convenience.

Solving the Problem

Above we have expressions for position and heading in the first three segments, we will split each equation into a y component and an x component and state them below:

(x,y)= rcos(3t1 +WCA)j - rsin(3t1 + WCA)i +wt1 + rsin(WCA)i -rcos(WCA)j

becomes:

(x)= - rsin(3t1 + WCA)i + wit1 + rsin(WCA)i = p1i

(y)= rcos(3t1 +WCA)j + wjt1 -rcos(WCA)j = p1j

And the equation of position for segment 2 which is:

(x,y) =- vt2fsin(3t1f + WCA)j - vt2fcos(3t1f + WCA)i+ wt2 + p1

becomes:

(x) = -vt2fcos(3t1f + WCA)i + wit2 + p1i = p2i

(y) = -vt2fsin(3t1f + WCA)j + wjt2 + p1j = p2j

The equation of position for segment 3 is:

(x,y)= rcos(3t3 + H2)j - rsin(3t3 + H2)i + wt3 + p2 + rd2

Which becomes: (note d2j becomes negative because the direction of displacement is downward.)

(x) = -rsin(3t3+H2)i + wit3 + rd2i + p2i

(y) =rcos(3t3 +H2)j + wjt3 - rd2j + p2j

Now we will add all the x and y expressions by using the full equations for p1 and p2.

(x) = rsin(WCA) - rsin(3t1f + WCA) + wit1f  - vt2fcos(3t1f + WCA) + wit2f - rsin(3t3f + H2) + wit3f + rd2i    (9)

(y) = rcos(3t1f + WCA)  + wjt1f - rcos(WCA) - vt2fsin(3t1f + WCA)+ wjt2f + rcos(3t3f  + H2) + wjt3f - rd2j  (10)

We can equate these two equations to the equation from segment 4:

(x,y) = 0j + vcos(WCA)i + w

Decomposed into directions:

(x) = vcos(WCA)i + wi

(y) = 0j

This gives us 2 equations, but there are three unknowns: t1, t2, t3 so we need a constraint equation, because heading ends where it begins (a total of 360° turned), and rate of turn is constant at 3°/s we can determine that:

t1 + t3 = 360°/(3°/s)

Therefore our constraint equation is:

t1 + t3 = 120s (11)

A successful attempt to solve this 3x3 system of equations was made using MATLAB. Here is a link to the MATLAB ".m" file that will solve for the required wind correction on the inbound and outbound legs of a hold: Link. This code will not run unless you have the Symbolic Math Toolbox for MATLAB, additionally the latest revision of MATLAB caused an error, will debug when time permits.

For those who are unfamiliar with MATLAB I will try to create an interactive web app in the future but for now the video at the top will be your best resource.

Pressure Altitude and Density Altitude

When asked what pressure altitude is or what density altitude is most pilots will inadvertently dodge the question and instead tell you how to calculate pressure altitude or how to calculate density altitude. This leaves unanswered the questions: What is density altitude? What is pressure altitude?

Here are the answers to those questions:

Dude, Pressure altitude is the altitude in the standard atmosphere at which you would find the pressure in question (usually the observed pressure).

We calculate pressure altitude for an airport by subtracting the current altimeter setting from 29.92 and multiplying the result by 1000. We then add this result to published field elevation

Example:

The altimeter setting in Prescott is 30.16 what is the pressure altitude?

Answer: (29.92 - 30.16) * 1000 + 5045 = 4805' MSL

This means that the pressure you are observing in Prescott will be found at an altitude of 4,805 feet in the standard atmosphere.

Now on to Density Altitude

I'm just gonna say it.
Density Altitude is the altitude in the standard atmosphere at which you would find the density in question (usually the observed density).

We calculate density altitude by correcting pressure altitude for non standard temperature using an E6-b (or something fancier).

To better understand Density Altitude, consider the following scenario, which actually happened once:

1. You are kidnapped by a tribe of five-legged aliens.

2. Using magical technology they suspend you at some point in the atmosphere and give you a magical density measuring device.
3. They ask you what your altitude is and place bets on how close your guess will be to reality.
4. They inform you that if you're more than ten percent off that they'll execute you by feeding you to the ravenous Bugblatter Beast of Traal.

...You read the output on the density measuring device and find ambient density to be .9 kg per meter cubed. You bust out your smartphone (which still has service) and follow the above links to a table of the standard atmosphere. You find that in the standard atmosphere a density of .9 kg per meter cubed occurs at about 3km altitude which is 3,000 meters high. You tell the aliens that you are 3,000 meters high. They are Imperial Aliens and demand the answer be given in feet. Being a very crafty pilot you know to multiply 3,000 by 3.28 to get feet. You bust out your smartphone again and multiply 3,000 by 3.28 to get 9,840 ft, you tell the aliens that you are at 10,000 feet MSL.
The aliens acknowledge that you are correct but decide to feed you to the Bugblatter Beast of Traal anyway. You die with a complete understanding of what density altitude is and how to calculate it.

Static Pressure and Dynamic Pressure

When explaining lift and various other aerodynamic and meteorologic phenomena it is vital to understand the difference between dynamic pressure and static pressure.

DYNAMIC PRESSURE
We feel dynamic pressure all the time, when we feel it, we call it wind. If you blow on your hand right now, you will feel dynamic pressure.

To get technical, dynamic pressure is equal to one half of density times velocity squared.

Where:

• q = Dynamic Pressure
• ρ = Density
• V = Velocity

Dynamic pressure acts in only one direction, the direction of the velocity.

STATIC PRESSURE

At sea level, static pressure is usually many times the strength of dynamic pressure but we rarely feel it. That's because the only things we feel are forces, and forces are caused by differences in pressure. And usually we are at the same pressure as the environment, which is about 14.7psi at sea level and about 12.2psi at 5,000 feet. If you are at sea level it's not just the air that's at 14.7psi, it's every cell in your body, it's every molecule that you are composed of that is at this pressure.

The immense power of static pressure, which unlike dynamic pressure acts in ALL directions, is only apparent when it interacts with a much higher or much lower pressure, such as the near zero pressure of space or the very low pressure experienced by aircraft cruising at high altitude. Below is a truly disgusting scene from the movie Alien (no seriously, don't watch it if heinous disembowelment grosses you out) where the immense forces created by static pressure acting against a vacuum suck the alien out of the spaceship. There are similar scenes in Star Wars and Star Trek but I couldn't find clips of them on YouTube.

Now it isn't only in gruesome space movies where effects of static pressure are demonstrated. If you've ever left toothpaste or contact solution or some other bottle of liquid in your car while traveling up to high elevation you've probably noticed the pressure escaping when you opened that bottle. Additionally if you have a sinus blockage or inner ear infection while travelling by car or aircraft you'll have felt that pressure in your sinuses or ears (which can be quite painful). Additionally if you've ever swam to the bottom of a pool you have probably felt the extra static pressure added by the water on your ears.

One last way to try to understand static pressure. Sea level pressure is 14.7psi, the average human adult has about 800 square inches on one side of their body, that means that if one side of your body had normal sea level pressure on it but the other side was somehow exposed to the vacuum of space that there would be a force of 11,760 pounds blowing you into space. You get 11,760 pounds by applying 14.7 pounds of force to each square inch of this average adult body. 

So there you have it. Static pressure and dynamic pressure, post questions and corrections below.

How a Wing Produces Lift (FAA Edition)

It can be a challenge to explain how lift is created while doing all of the following:

1. Use Bernoulli's Theorem (which is what the FAA likes).
2. Avoid telling obvious falsehoods (which is what flight instructors like).
3. Be vaguely comprehensible.

Below is one explanation which meets all three criteria.

--------------

Because of the shape of the wing and the Angle of Attack, air flows faster over the top of the wing than over the bottom of the wing. In this sentence is hidden all the complexity and mathy nonsense that scares people away from looking into how lift is created, I'll re-state it. Because of the shape of the wing and the Angle of Attack, air flows faster over the top of the wing than over the bottom of the wing.

Now let's talk about types of pressure. Total pressure, is always equal to dynamic pressure plus static pressure (in subsonic ideal flow) ...which is illustrated by the below equation.

Now dynamic pressure is proportional to velocity, so if velocity goes up, dynamic pressure goes up. As we stated above, air flows faster over the top of the wing than over the bottom of the wing which means there is greater dynamic pressure on the top of the wing than on the bottom

Because total pressure must stay constant, the higher dynamic pressure on the top means static pressure must be lower on the top. Conversely, the lower dynamic pressure on the bottom means that static pressure is higher on the bottom.

Dynamic pressure only acts in one direction, the direction of the arrows above and below the wing, which means it does not exert any force on the wing. This means that only Static Pressure exerts a force on the wing.

So only static pressure exerts a force on the wing, and dynamic pressure is higher on the top of the wing than on the bottom of the wing ...which means that static pressure is higher on the bottom of the wing than on the top of the wing. This is lift. Lift is the difference in static pressures between the top and bottom of the wing.

For a more complete explanation watch this lecture. 

The True Story of P-Factor, for Pilots

P-Factor, or Propeller Factor (or asymmetric blade effect or asymmetric disc effect), is an important aerodynamic phenomena for pilots to understand, at least it is if your plane has a prop.

P-Factor is often explained to pilots in silly ways using false analogies (No! the blades do not have mouths, one does not take a bigger bite of air than the other!). At AirCrafty we absolutely detest false analogies and believe that anyone using them should be mailed third class to a dark place between two stars. What follows is the True Story of P-Factor:

-------------------------

Below is an image of an aircraft whose 2-bladed prop has one blade (the descending blade) coming straight out of the screen and the other blade (obviously the ascending blade) going back through the screen. The dashed lines going through each blade represent the chord lines of each blade.

Angle of Attack (AoA), as all good pilots know, is the angular difference between the chord line of an airfoil and the relative wind. If the above prop began spinning while the aircraft was stationary on the ramp the Angle of Attack (AoA) would be the same as the angular difference between a vertical line and the chord line as shown below:

But if the aircraft were moving forward, finding out where the relative wind was coming from would be a little tougher, we'd have to add the velocity of the aircraft to the velocity of the prop as shown below:

Don't be intimidated by the busy graphic, stare at it for five minutes and it will make sense (...because the aircraft is moving forward the wind hits the prop from a more forward direction reducing the angle of attack on the prop blade by an amount equal to the BHA). 

Bonus! BHA stands for "Blade Helix Angle" a term you can use to impress some people at the public pool later today (the blade helix is traced by the prop tips of this C-130).

Now go watch this 3 minute YouTube video, the guy's accent is great and it'll help you solidify your grip on things before we move on.

OK so far we have an angle of attack on the blade but no P-Factor. Now we'll pitch the aircraft up and continue to fly straight and level (neither climbing nor descending) ...slow flight style; as we do this, P-Factor will emerge in mysterious fashion like consciousness emerging from a brain.

You've probably already gazed furtively down at the below graphic. Be intimidated by this busy graphic. Panic, hyperventilate, go through the five stages of grief, then recall the Nietzschen aphorism your dad taught you. Look! the busy graphic didn't kill you! Therefore you're stronger. With this new strength you will now understand P-Factor como un jefe.

There's a couple things going on here:

1. Because we pitched up, the velocity of each blade is no longer perpendicular to the velocity of the aircraft. This means a large component of the aircraft's velocity adds to the descending blade's velocity. For the ascending blade there is now a component of the aircraft's velocity that subtracts from the blade velocity. The addition of a component of the aircraft's motion to the descending blade and subtraction of a component of the aircraft's motion from the ascending blade is the first part of P-Factor. I left the old aircraft velocity vectors that are perpendicular to the blade velocity vector on the above graphic and made them a faint green color so you can still see how things were before we pitched up.

2. I also left the old relative wind vector for the descending blade on the above graphic (but left it off for the ascending blade) and made it a faint blue color, it is mostly hiding behind the new relative wind vector of the descending blade. Notice that the old relative wind vector isn't just shorter (and therefore the relative wind is slower) but it's also at a slightly different angle than the new relative wind vector (the not-faint blue arrow). This new relative wind vector is closer to vertical which means the BHA has been slightly reduced which means the angle of attack has been slightly increased. On the ascending blade the change in angle of attack is negligible in most cases (more on this in the Technical Section below). The increase in angle of attack on the descending blade combined with the negligible change in the angle of attack on the ascending blade is the second and final part of P-Factor.

I'll re-state it all together here...If the relative wind is not perpendicular to blade velocity, a component of the aircraft's velocity adds to the descending blade's velocity and subtracts from the ascending blade's velocity; at the same time, the angle of attack on the descending blade increases while the angle of attack on the ascending blade changes a negligible amount. The descending blade therefore has greater velocity and greater angle of attack than the ascending blade, therefore it creates more thrust than the descending blade, this asymmetric production of thrust causes a yawing moment (a yawing moment which is normally to the left and needs to be counteracted with right rudder), this yawing moment is P-Factor. 

And that, is the true story of P-Factor.

If you want to be able to quantify the affects of P-Factor rather than just understand it conceptually, and if you want to learn what "PAAoA" stands for, then continue on to the technical section below.

-------------------------

PAAoA stands for Prop-Axis Angle of Attack. It is the angular difference between the axis about which the prop spins and the relative wind. The PAAoA, in combination with aircraft velocity and prop velocity, determine the magnitude of the moment we call P-Factor. The relationship between these three quantities is shown below:

The black dashed line is vertical, the relative wind (RW) velocity can be calculated using the Law of Cosines, C^2 = A^2 + B^2 - 2ABcos(c) modifying this for the present triangle and solving for relative wind gets us:

Once we have relative wind the BHA can be calculated using the Law of Sines. I won't bore you with the Law of Sines, here's the resultant equation solved for the BHA:

Now I'd like to curse Blogger four times for not building an equation tool into blogger and constantly monkeying with settings causing mathjax and various other LaTeX readers to be unreliable. Curse you Blogger, Curse you Blogger, Curse you Blogger, Curse you Blogger.

 $E=mc^2$.

UNDER CONSTRUCTION